Monthly Archives: August 2008

Tessellations

Tessellations with figures

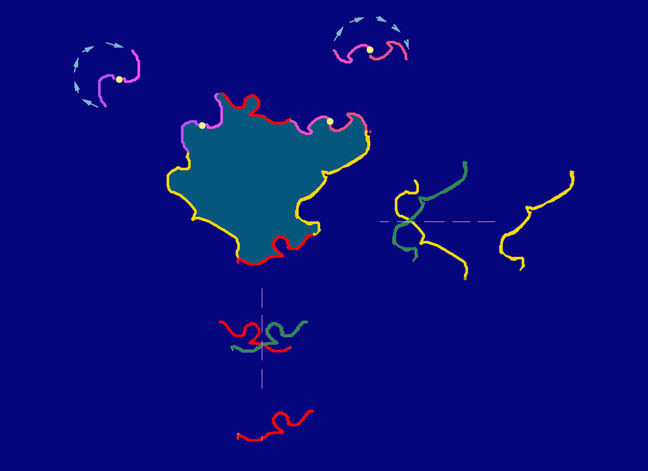
A tessellation is a pattern made up of elements that repeat with no gaps and no overlaps.  The elements may be abstract shapes, or may be recognisable objects or creatures, like the ones in the tessellations of M.C.Escher.  When I begun playing around with tessellations, I thought understanding the procedures needed to make patterns that tessellate would be the hard part.  I thought it would be fairly easy to find creatures in whatever shapes I ended up with.  Not so.  The procedures aren’t so hard.  But fitting creatures into them I found really difficult.  Here’s one of my first attempts.  I started with one of the most complicated recipes for a tessellation.  

 
 

For the details of the procedures, which give shapes that tessellate, see my tessellation tutorial. Essentially, the boundary of every shape that will tessellate is made up of pairs of lines. Within each pair, an identical line repeats, either by rotation, reflection, or just by shifting over. In the example above, there are four pairs of lines, two of them with rotations and two with reflections.

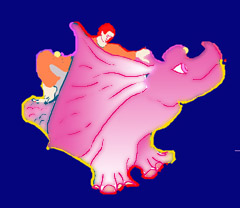
But what creature could I discover in this shape? Here’s what I came up with, a cross between an elephant and a rhino, with a little man on its back.

And it does tessellate!  It gives a pattern in which the elenoceros repeats four times, right way up facing both ways, and then upside down facing both ways.

Ambiguous images, illusions and aesthetics

Ambiguous patterns

I reckon some images look beautiful because they bamboozle the brain processes we normally depend on to make sense of the world.  I don’t know why  that can help make patterns and pictures look beautiful. Nor do I think perceptual puzzlement is the essence of art, or anything like that.  But just from a practical point of view, if you are an artist (or a composer, poet or architect), a motif that’s puzzling can seem to offer a stepping off point for aesthetic effects.

Here are two beautiful examples from architectural decoration, both just about 500 years old.  The first is the dome of the Mausoleum of Sultan Qaitbay in Cairo.

What’s puzzling about this is that a single line segment can be part of the edge of an object, such as a star, and at the same time part of a line that meanders over the whole surface.  Edges don’t behave like that in everyday vision.  Here’s the dome with added lines, left below, to show what I mean. Look at the segment that is labeled with both blue and yellow lines.

Then note that you can do just the same with the lines that outline the octagons on the ceiling in the picture to the right – every edge is also part of a fan of lines.  That ceiling is in Christchurch Cathedral in Oxford, and we even know who designed it – William Orchard, the Master Mason.  Now we’d call him the architect.  Here’s a picture showing a bit more of the ceiling.

I don’t think it’s just the puzzling features that make these patterns so beautiful.  Interlace patterns like these look like small segments of patterns that go on for ever, and in both Christianity and Islam were a metaphor for perfection and heaven.  And that’s how I reckon artists turn perceptually puzzling effects into something more than amusing images – they choose motifs that are also metaphors for some deeper meaning.

You may be more familiar with interlace as a technology for managing video images.  For interlace as a pattern motif in christian art, see the Wikipedia article on Celtic Knots.  Or try here to find out about interlace patterning in the context of islamic faith.

Ambiguous images, Illusion demos with animations, Optical Illusions

Revolving Heads

3 Comments

Heads that present one character one way up and another when rotated have been favorite illusions for over a century. Here are two heads from a cartoon story I devised about a boy who gets stuck in a weird hotel. The receptionist and chef, (Mr. and Mrs. Turner …. ) seem OK at first, but then transform into two sinister old men when their heads rotate.

For an animation of Mr. and Mrs. Turner see below:

There are loads of great rotating heads at:

http://members.lycos.nl/amazingart/E/6.html Rotating Heads

Soap bubble pictures

Soap Bubbles

Soap bubbles aren’t illusions, but I am fascinated by them, and have a special technique for photographing them, (with a little help from Photoshop).

Here’s a picture of a bubble moon over London:

I’ll return to the subject of bubble pictures. Meanwhile, the most beautiful images of soap films are made by Karl Deckart.  Jason Tozer is another photographer who has recently made some stunning new photos of soap films and planet like segments.

Impossible worlds (inc. staircases), Optical Illusions

Optical Illusion Cartoon Story

For years I’ve wished someone would make an animated cartoon in which the events depend on the kind of visual transformations we see in many illusion pictures. It won’t be easy. Salvador Dali loved effects of these kinds, and helped sketch out a scheme for a Disney movie (though not one with a real storyline) in 1945/6. It’s called Destino. It didn’t get made, until Disney’s nephew Roy Disney made a version in about 2000. I don’t think it was so successful, but it was a fascinating chance to see what works, and what is less successful when animated. Take a look at a trailer and decide,

http://www.youtube.com/watch?v=iO1ghQFSXro

I reckon Goo-Shun Wang’s wonderful, recent animation of a character trapped on an Escher-style, never-ending staircase is far more successful:

http://www.moillusions.com/2007/04/halluciis-problem-illusion-video.html

To explore the kind of effects I think might work in a narrative, I devised a couple of still-picture cartoon stories. Here’s a pair of frames from one you can view on the www, in which the characters are almost trapped on another never-ending staircase, when a spiral stair suddenly transforms:

Check out the whole thing at:

http://www.Opticaloctopus.com

it also includes loads of hints on drawing illusion pictures.

Geometric illusions, Optical Illusions

(Not so) Geometric Illusions

Many of the illusions in popular books are geometric ones, in which lines that are really parallel look wonky, or lines that are aligned seem not to be. Most of these figures were discovered by German researchers, a hundred to a hundred and fifty years ago. But how geometric do they have to be? With graphics packages it’s easy and fun to explore. Here are versions of two famous illusions, one showing apparent divergence where the other presents convergence, against the same “zebra skin” background.

Continue reading →

Impossible worlds (inc. staircases), Optical Illusions

Escher’s “Waterfall” Explained

Here’s a demonstration of one of M.C.Escher’s famous pictures, the Waterfall. (Just put Escher Waterfall into Google Images to see his version).

First of all, you need to understand how a famous “impossible figure” called the tribar produces its effect.

One the left, in the picture above, we see the tribar as an impossible figure. The top of the vertical bar to the left seems to be at both the nearest point in the image and the furthest point at the same time. In the image to the right, seen from a slightly different viewpoint, there’s no problem. The top of the vertical bar really is nearest to us. But seen as to the left, with the arm exactly aligned with the end of the arm to the rear, our brains go for the option that bars are connected as the most probable configuration – even though it’s impossible.

If you are good at fusing stereo picture pairs without a viewer, you’ll find these two images will show the tribar in 3-D.  For a guide to how to view 3D picture pairs without a viewer, in “cross-eyed” mode, try:

http://www.3dphoto.net/text/viewing/technique.html

There are other sites if you search on “viewing 3d picture pairs” or similar, and also animated guides on Youtube.

Now for Escher’s Waterfall. On the right below is my stripped down version. The water seems to be flowing uphill, and then pouring down to the bottom again. But then compare the right hand image with the small middle image: the configuration is just two tribars, one on top of the other. And on the left, with the vertical posts sawn off, so that our brains don’t have to connect them to the zig-zagging channels, the whole configuration seems to recede horizontally as it should, instead of stacking up impossibly.

Note added 16/5/17:  There’s a brilliant movie demo of this on Michael Bach’s illusion site.